A comparative analysis of pedaling in seated versus standing cycling
by Martin Krause 2004
Task Demand
Cycling requires efficient pedaling action to maintain adequate power output to win a race. Importantly, an endurance sport such as cycling requires a pedaling action, which provide sufficient cadence to maintain a steady state of efficiency. In particular race advantage over competitors can be achieved through efficient hill climbing. Anecdotal evidence suggests that cyclists prefer to stay seated as long as is possible before rising out of the saddle. However, acceleration during hill climbing is frequently achieved through standing off the saddle. This acceleration appears to be achieved through the use of body weight and arm moments as well as increased use of shank and thigh movements. Therefore, increased plantar flexion and hip-knee extension may be expected.
One of the key determinants of muscle co-ordination and movement efficiency during cycling is pedalling cadence. Such cadence needs to occur across multiple joints and limb segments. Whereas, inverse dynamics requires changes in velocity to achieve accelerating body parts. Optimal pedal cadence for long distance cyclists tends to be between 85 and 95 revolutions, in order to achieve minimal neuromuscular fatigue. In contrast, maximum power is achieved with pedal cadences of between 110-135 rpm. Higher pedal rates lead to shorter duration pedal cycles, coupled with longer duty cycles, recruitment of fast twitch muscle fibres and de-recruitment of slow twitch muscle fibres leading to significant inefficiencies and loss of power at higher cadence. Furthermore, people with long limbs aren't able to develop the same moments of inertia compared to shorter limbed people. Chaos theory would suggest that the randomization of movement pattern requiring pertubations of periodicity may make the scaling of muscle action/inaction important when considering minor fluctuations in cadence and moments of inertia. Three orthoganal axis with a fixed point of pertubation, a moving point of seemingly random pertubations and everything else. Only in this way can a supposed cadence of supposedly constant velocity be used to describe inverse dynamics i.e. non-linear mechanics to describe biomechanics. As such the intrinsic firing pattern (motor unit potential shape, firing rate variability or lack there of, coherence between motor units) of muscles need to be established, as well as their co-ordinated firing pattern across the same muscle, other muscles on the same body segment and muscles of different body segments executing the same motor task. A term entrotropic half life (EnHL) using EMG was coined and developed to examine what limits mechanical power output at higher cadences above 120rpm. Hodson-Tole et al (2020, Med Sc Sp Ex, 52, 1, 214-224) found that, in trained male cyclists (~ 10000km/yr, average 33yrs), changes in the individual muscle excitations can occur even when changes in co-ordination were more limited. Importantly, they demonstrated that features of the EMG change in a non-linear fashion with incresing cadence and that both 80 and 120 rpm represent inflection points, hinge points or potential manifolds critical in EnHL relations. In other words, at cadences less than 80rpm and higher than 120rpm may have fewer fluctuations in muscle recruitment or firing characteristics leading to reduced application of force at the pedal. This variability is particular true for the bi-articular muscles, such as the gastrocs (medial and latral), biceps femoris, rectus femoris, whereas the vastus medialis and vastus lateralis reached a minimum at 80rpm and did not change across the faster cadences studied.
When considering moments of inertia it is probable that efficient cycling technique involves the ability to maintain saddle contact even during hill climbing. Additionally, when considering the sliding filament model of muscle action, it is likely that due to specificity of muscle contractile action it will be the cyclist who can maintain a pedaling action which does not vary considerably from sitting to standing who will be most efficient in hill climbing action. However, power may be a greater consideration than efficiency when standing out of the saddle. Therefore, effective pedal force may also be the result of arm action. Finally, when comparing body position from the seated to standing position, the cyclists center of gravity moves further forward and therefore more likely to be over the center of crank rotation in the standing position.
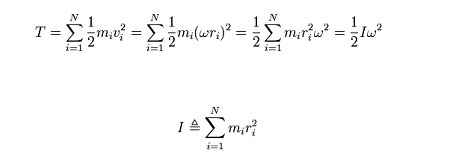
Introduction
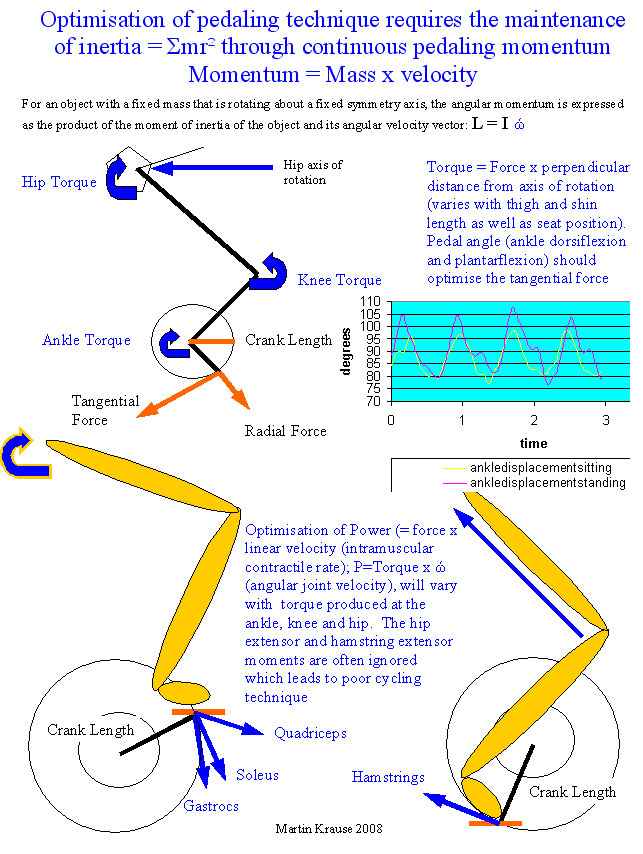
Efficient pedaling requires the effective transfer of energy from the lower limbs into the pedal. Consequently, this energy is transferred into the crankshaft, which drives the chain that accelerates the bike. Importantly, the effective use of force, through the pedal at the correct point in time of the crank cycle, is required. Anecdotally, this force is thought to be applied perpendicular to the pedal crankshaft during the down stroke. However, the foot is seldom perpendicular to the pedal crankshaft (Cavanagh et al 1986). Additionally, as the cyclist moves from the seated to the standing position, logic would dictate that the pedal – crankshaft angle will change and the range of the down stroke where maximum force is applied will also change. Finally, since the pedaling action is a cyclic activity then the recovery phase (upstroke) of cycling requires efficient use of energy through the inertial forces of the pedaling action (Cavanagh et al 1986). However, in standing these inertial forces change as the joint torque changes. Therefore, energy can be lost through incorrect pedaling angle resulting in reduced effective force.
Literature Review
Investigations examining the kinematic parameters of seated versus standing cycling are sparse. Most studies have focused on cycling in level terrain (Caldwell et al 1999). Why cyclists adopt the standing position remains unresolved (Davison et al 2000, Ryschon et al 1991). However, the inertial properties of cadence and quasi static forces of lower cadence may be the deciding factor as to whether a cyclist determines the need to stand. Lower cadences would appear to be less efficient and therefore would require greater force to be applied through the pedal (since Power = Force x velocity). Indeed, peak resultant pedal force and peak crank torque increased by 200% and 130% respectively in the uphill standing position (Caldwell et al 1999). Stone and Hull (1993) suggested these forces to represent greater than 60% body weight in the standing position. Furthermore, these peak forces occurred later in the crank cycle for the standing position (at 155 degrees for force and at 130 degrees for torque) than in the sitting position (100 degrees for force and 85 degrees for torque) (Caldwell et al 1999) (see Figure 1). Interestingly, the higher peak kinetic values were linked to kinematic changes in pedal orientation and therefore changes to force vector direction throughout the crank cycle (Caldwell et al 1999).
Investigation into kinematic parameter variations between various seat heights suggest that the peak knee extension increases from 65 to 125 degrees in the low seat to 25 to 105 degrees in the high seat configuration. Additionally, ankle angle varied from 5 dorsiflexion (DF) to 25PF degrees in the low seat condition to 12 plantar flexion (PF) to 20PF degrees in the high seat condition. Peak knee extension and flexion occurred at 170 degrees and 350 degrees of the crank cycle respectively (Gregor et al 1996). This suggests that at least in the varied seated position the muscles length tension relationships would change, thereby affecting force capabilities of the muscle by placing them in a different area of their length-tension and force-velocity curves (Gregor et al 1996). Extrapolation of these results to the standing position, suggest similar implications for the length-tension and force-velocity relationship for the production of power during uphill climbing. However, hip and knee angular kinematics have opposing effects and therefore result in minimal muscle length change in the hamstring muscle (Rugg et al 1987). A similar pattern was seen in the gastrocnemius muscle. In contrast, changes in the length-tension relationships of the mono-articular muscle such as soleus may be expected as it does not cross both the knee and ankle. The magnitude of initial lengthening was about the same in the various seated positions, however the magnitude of shortening increased dramatically from about 1cm to approximately 2.5cm (Rugg et al 1987). Therefore, an expected kinematic outcome for the standing position is increased plantar flexion. This should result in an increased rate of shortening of the soleus muscle from it's initially lengthened position.
Impulse occurs earlier in the crank cycle than does the development of the effective pedal force (figures 1 and 2). Therefore, an increased rate of muscle shortening to accommodate the application of muscle force over an increased range of motion may be seen through altered velocity and acceleration at the ankle joint. Paradoxically, the soleus muscle is considered to be an endurance muscle with slow twitch properties suggesting lesser abilities to shorten quickly (Soderberg 1992). Furthermore, muscles with a large proportion of it's myofilaments in series are considered to have a greater propensity to shorten with greater velocity over a greater range of motion. However, the soleus muscle has a large proportion of it's myobrils in parallel (Jones et al 1990). Additionally, the soleus muscle has some of the largest angles of pennation (up to 60 degrees) seen in the human body (Soderberg 1992). Large angles of pennation reduce effective force transmission (Lieber 1992). Consequently, the implications of altered loading of such an essentially slow twitch endurance muscle are either changes in the myofibril architecture with training, a reduction in cadence in the standing position, altered timing in the application of torque or synergistic muscle load sharing (Caldwell & Li 1998).
In order to examine changes in joint kinematic parameters we maintained a constant pedaling frequency. Additionally, the effect of load sharing between the soleus and gastrocnemius muscle were examined by looking at the kinematic parameters at the knee and ankle to see if they would result in little change in the length of the gastrocnemius muscle, as is suggested by the literature (Gregor et al 1996). Finally, examination of the ankle joint angle (see figures 3 and 4) during the crank cycle should determine variations to the timing in the application of effective force and therefore torque during the crank cycle, described by Cavanagh et al (1986).
Fig 1: pedal impulse: Since Impulse = force x time, it can be seen from the light shaded area that 50% of the propulsive impulse is delivered between 60° and 120° after TDC (top dead center)
Fig 2: pedal effective force: the light shaded area represents ‘unused force' whereas the dark shaded area represents negative effective forces. The objective of the rider is to reduce the size of the unused force and eliminate the negative effective forces without decreasing the size of the propulsive impulse
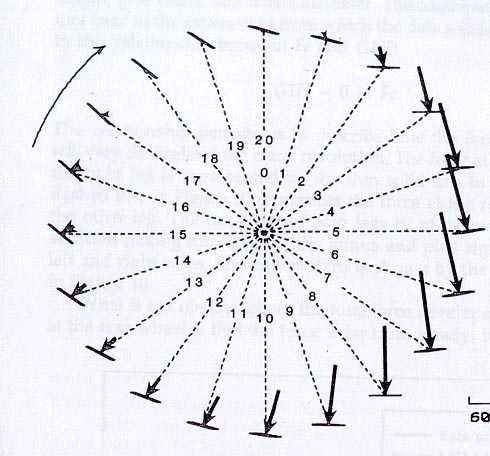
Fig 3: pedal angle and timing of torque: The orientation of the pedal and the resultant force vector are shown at 20 positions of the crank cycle. It is interesting to note the orientation of the force vector during the first half of the revolution and the absence of pull up forces in the second half.
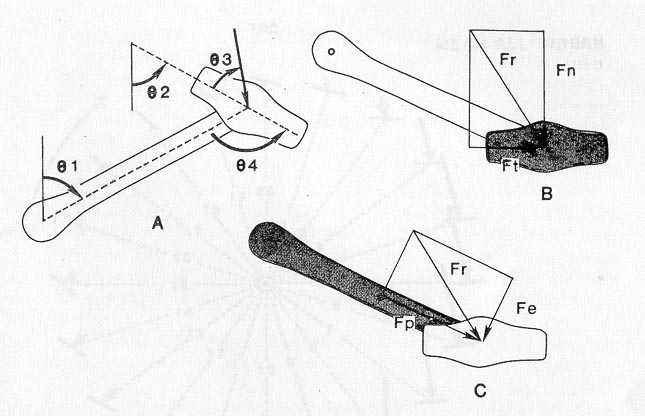
Fig 4: pedal angle: A shows the angle conventions used for the calculation of force. B and C show the resultant force. Fe is called the effective force because it represents that component of force that is effective in generating a force at the rear wheel.
(Figures 1-4 from Cavanagh et al 1986, pp103-109)
Methods
The subject was a healthy 37 years old male amateur cyclist. He was asked to cycle at 60 revolutions per minute in the sitting and standing positions using a bike ergonometer. He had markers placed on the lateral aspect of the shoe at the region of the 5th metatarsal, at the lateral maleolus, at the superior head of the fibula, and at the greater trochanter. A digital video camcorder (Canon Pal MV530i) recording at 50 frames per second was used to record the cycling action. Recordings were made during steady state 60 revs per minute cycling.
The digital video was then captured on the computer using the Ariel Performance Analysis System (APAS) system of kinematic analysis. The captured film sequence was trimmed to remove unwanted sequences, digitized, transformed using the calibration points, and filtered cut off frequency to smooth out errors in digitizing. Once the results were displayed, the data was cut n' pasted into an excel spreadsheet and then graphed for comparative qualitative analysis.
Presentation of results from kinematic analysis
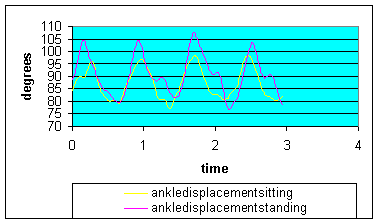
Graph 1: Ankle angular displacement: ankle displacement in standing demonstrated considerably larger (approx 8 degrees) plantar flexion.
Graph 2: Ankle angular velocity: ankle velocity into plantar flexion during standing was somewhat higher, however the change of direction into dorsiflexion was more pronounced in the standing position
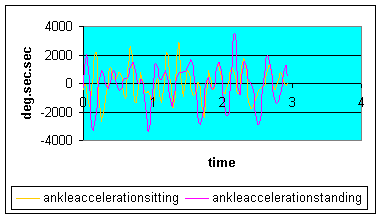
Graph 3: Ankle angular acceleration: ankle acceleration into dorsiflexion (top of graph) appears on average higher than the acceleration in plantarflexion (bottom of graph) during the standing position. This result may be expected as the total displacement of the ankle has increased for the same revolutions per minute. Therefore greater ankle displacement must occur in the same amount of time. Additionally, greater acceleration may be synonymous with greater impulse generation (I=F.t=m.a.t=m.v) and therefore greater momentum. However, the momentum generated will be influenced not by velocity alone but by the greater mass of body weight in the standing position.
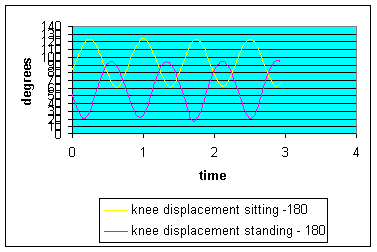
Graph 4: Knee angular displacement: The total range of knee motion did not change significantly from the sitting to standing positions. However, the displacement occurred in a more extended position (bottom of graph) of knee extension in the standing position. Yet the results in the standing position are within the expected range of motion if the seat height is correctly fitted for the rider (see below).
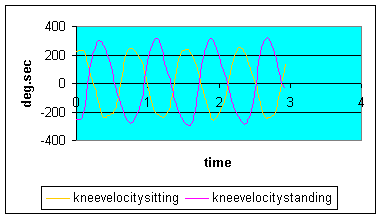
Graph 5: Knee angular velocity: Knee velocity did not vary greatly between sitting and standing as the displacement between the two positions were similar.
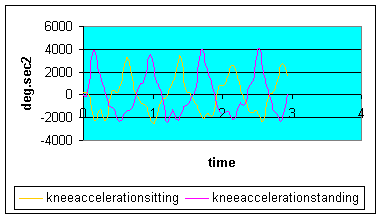
Graph 6: Knee angular acceleration: Knee acceleration did not vary greatly between the sitting and standing positions suggesting that the Impulse generation and momentum were similar at the knee joint in the 2 conditions. These results suggest that a greater impact may be expected on the internal mechanics of the actin-myosin sliding filament in the quadriceps and hamstrings muscle due to their working in a different range of motion. Importantly, since mass is increased through the application of body weight in the standing position, then the velocity of shortening may be expected to decrease.
Discussion of results
Our results suggest that ankle displacement is strongly influenced by changes in body position. The results demonstrate an average range of ankle displacement of 25° (range = 80-104° ) for the standing position and 17° (range = 80-97° ) in the sitting position. Similarly, Caldwell et al (1999) demonstrated 17° of ankle displacement in standing. This compares with 40° 58'+6.01 and 25° 7'+14.12 in sitting and standing respectively found by Shemmell & Neal (1998) and Too (1996). These authors provided normative data suggesting that the range of angular displacement at the ankle in standing was 137° 40'+9.53 to 96° 82'+11.07, with the pattern of angular displacement showing a trend to reach maximum plantar flexion earlier in the pedal cycle in the non-elite group (Shemmel & Neal 1998). This suggests that the optimal pedaling angle during standing is always in some plantarflexion thereby providing an effective force angled downwards and backwards. Therefore, during the recovery phase of the pedal cycle little or no upward pull can occur on the pedal. When considering Figures 1 and 2, this technique should then minimize negative effective forces described by Cavanagh & Sanderson (1986) on the upstroke of the pedal cycle. However, prolonged plantarflexion does suggest an increase of ‘unused force' at the bottom of the pedal cycle. Our results vary significantly as our subject demonstrated the same amount of dorsiflexion whether in sitting or in standing. Additionally, this dorsiflexion occurred below the 90° angle suggesting a large amount of eccentric calf muscle activity prior to plantarflexion. In contrast, data from Caldwell et al (1999) demonstrated that regardless of the condition, ankle moment profiles demonstrate exclusively plantarflexor torque throughout the crank cycle, with the highest values after 90° , i.e. in the latter part of the downstroke. Shemmel & Neal (1998) concluded that ankle angles were found to be the best discriminative tool as the ankle plantarflexors contribute more to force production in the standing versus seated position.
Our knee displacement results in standing of 72° and 62° in sitting contrasts significantly with 28° 69'+8.80 and 73° +6.41 (Shemmell & Neal 1998). Caldwell et al (1999) demonstrated knee displacement of 82° 39', with a range from 17° 6' knee extension to 99° 45' knee flexion in the standing condition. Shemmel & Neal (1998) attributed their dramatic change to the forward shift of the cyclist over the pedals during standing. The significant difference between our results and their results may be due to the outdoor 10.5% incline condition, which they used for their analysis. Additionally, they factored the bicycle tilt in the frontal and saggital planes into their calculations. Stone & Hull (1993) suggested a 5° phase lag due to the 8% incline used in their investigation. Finally, Caldwell et al (1999) had their subjects use their own bicycles and were asked them to complete the task as closely as possible to real racing conditions. These latter factors are in contrast with our protocol as we maintained the same incline and cadence; whereas in racing conditions the incline increases and cadence frequently decreases during the uphill phase of the race (82rpm to 65rpm {Caldwell et al 1999}). A notably exception to this rule was an increase in Lance Armstrong's cadence during the 2001 Tour de France on Col Du Madelaine.
Effectiveness of performance
The demonstrated kinematic changes suggest kinetic consequences to work and power production. Caldwell et al (1999) demonstrated an increase in the peak magnitude of the ankle plantarflexor and knee extensor moments during uphill cycling. They suggested that these changes were likely related to the total work done per crank revolution as a consequence of holding power output constant while cadence is decreased.
Our kinematic results tended to suggest that peak plantar flexion occurred earlier in the crank cycle. Yet the results obtained by Caldwell et al (1999) suggest distinct kinetic modifications at the knee and ankle later in the crank cycle in the uphill standing position. Ankle plantarflexion moment increased by 160% and shifted by roughly 45° in the crank cycle. The knee extensor profile also showed a shift towards the late downstroke period, with a bimodal extensor pattern that exhibited a second peak at about 135° crank angle (Caldwell et al 1999). In the uphill standing posture the cyclists body moves forward and upward. The knee extensor phase persists into the first portion of the upstroke, as does the ankle (Caldwell et al 1999). These kinematic changes result in altered pedal orientation to a more toe downward position throughout the crank cycle and modification of the applied pedal vector (Caldwell et al 1998).
Features which contribute positively to performance
In the standing position, the line of action of the force vector is closer to the ankle joint center, but the pedal force is much larger resulting in an ankle joint moment which is increased in the later part of the downstroke. Similarly, in the standing position the force line of action is posterior to the knee in the earlier part of the crank cycle (140° ), but moves in front of the knee later in the downstroke (near 160° ) (Caldwell et al 1999). Unfortunately, we were not able to establish this point because we did not use a body marker in our analysis.
In standing the removal of saddle support results in the greater contribution of gravitational forces. In the later part of the downstroke, the rider can make greater use of the effective force by angling the pedal downwards thus increasing the backward horizontal component of the force. In contrast, in the seated position, the backward horizontal component can only be generated by pulling back on the pedal with a flexor moment (Caldwell et al 1999).
Stone & Hull (1993) demonstrated additional positive power moments through the action of the arms during standing. The arms pulled up and back during the power stroke of the corresponding leg and pushed down and forward during the upstroke. This represents a reversal in direction when compared to the sitting condition. Importantly, the maximum lean of the bicycle corresponds with the maximum power output at about 140° of the crank cycle (i.e. downstroke phase). Because the arms pull up and back at this instant, the normal force would be affected. Therefore, achieving forces greater than body weight is a result of the action of the arms.
Improvements, which could be made to performance
Based on inverse dynamics analysis assumptions have been made that mono-articular muscles contract in the absence of antagonist contraction. However, several authors suggest that mono-articular and bi-articular muscles play different roles in the production of multi-segmental actions such as cycling. In particular, the uphill standing position, which frees the hips from the constraint of the saddle, may play an important role in the development of efficient propulsive forces when cycling uphill. Therefore, conclusions based on inverse dynamics may be less accurate when examining the standing condition.
Changes in the effective pedal force direction due to alterations in body position and therefore joint position suggest alterations in the functioning of bi-articular muscles. The gastrocnemius, rectus femoris and hamstring muscles have been shown to be active during cycling (Li & Caldwell 1998). In particular, the later muscle has been attributed to providing a hip extensor moment through a backward pull on the femur through it's insertion into the pelvis (Lombards paradox). Based on kinematic and kinetic analysis, Fregly & Zajac (1996) concluded that the net ankle and hip extensor joint torques function synergistically to deliver energy (38 joules = 50-82% of energy generated to the limb) to the crank during the downstroke. However, they attributed these net moments to the mono-articular soleus and gluteus muscles. Mono-articular muscle may indeed provide the majority of muscular work at each joint, yet the bi-articualr muscles probably redistribute the energy across adjacent joints (Caldwell et al 1999).
During the upstroke, contrary to popular opinion an ankle-hip flexor synergy is not used. Rather, the ankle extensor torque transfers energy from the crank to the limb in the upstroke (Fregly & Zajac 1996). Suggestions were made that in the presence of increased force through application of body weight in the standing condition that the two energy sources (gravity and ankle extensor torque) could restore the potential energy of the limb in the upstroke (Fregly & Zajac 1996). Therefore, the standing position may provide a more effective lower extremity joint angle that increases effective force production (Too & Landwer 2000). However, it would appear more likely that the effective pedal forces are enhanced by positive torques developed by the arms during uphill standing through the pull upwards on the handlebars during the downstroke and the push forward on the handlebars during the subsequent upstroke (Stone & Hull 1993). The maximum upward pull on the handlebars corresponded with maximum power production at 140° of the crank cycle (Stone & Hull 1993).
Minimizing the joint moment cost function would minimize leg stress and fatigue, thus maximizing propulsive power and cycling performance. However, based on the muscle force-velocity relationship, maximal power is not achieved through high velocities and low loads, nor low velocities and high loads, but rather a moderate load and velocity (Winter et al 1996). The standing condition does not suggest optimization of performance due to the high load and low velocity often employed by cyclists. Suggestions that the bi-articular muscles may be optimizing their length tension relationship in the standing condition don't appear to be supported by the literature. Instead, the bi-articular muscles are thought to act as ‘energy straps' transferring energy from one limb segment to another (van Ingen Schauer 1989). Furthermore, the biarticular muscles are considered to control the direction of applied external forces by balancing the relative moments of the adjacent joints they cross (van Ingen Schauer et al 1992). Importantly, bicycle lean and effective force production by the arms (as demonstrated by Stone & Hull 1993) have important implications for the transfer of energy through the body to the pedal.
Why particular features of the task may or may not be beneficial
Anthropometrical differences between cyclists, suggests that the taller cyclist with longer leg lengths (and greater inertial properties) would minimize their kinematic moments through reduced pedaling cadence. Conversely, the shorter cyclist (with shorter leg lengths and lower inertial properties) would minimize their quasi-static moments with a higher pedaling cadence (Too & Landwer 2000). Regardless, a cyclist is more likely to stand up out of the saddle to maintain their inertial properties of cadence through a change in joint moments as well as the addition of body weight to the limbs inertial forces. Additionally, the arms provide considerable positive moments during the power phase of the crank cycle. These moments allow the pedaling force to exceed body weight (Stone & Hull 1993). However, unless there is sufficient neurophysiological control and co-ordination, these increased forces may not contribute to effective pedal force. The bi-articular muscles are thought to not only transfer energy but also control the direction of force production. As stated early the standing position results in a body position almost vertically over the center of rotation of the crank, which may be a means to control the perpendicular distance from the point of force application to the axis of rotation. Hereby, the cyclist may attain greater effective force through the pedal. However, due to the use of the arms greater energy is expended which suggests reduced efficiency as less amount of time can be spent in the standing position due to finite amounts of energy (ATP). Therefore, ideally the cyclists should adjust their seat height in order to obtain minimum kinematic joint moment cost function (Gonzalez & Hull 1989). Tri-athletes tend to have bicycles built whereby their seated position is more forward. They also have the additional benefit of being able to slide the seat forward on the seat post thereby bringing their body closer to the center of the cranks (Price & Done 1997) without the need to stand up out of the saddle. However, in contrast to cyclists, triathletes need to maximize their efficiency in swimming and running as well. The forward seating position may more resemble to kinematics of running thereby opitimising the specificity of training the myo-filaments. Additionally, reduced need to use the arms after the swimming leg may represent better cost efficiency in already fatigued muscles.
Conclusions
Our data demonstrated greatest change in the kinematic parameters at the ankle joint in the sitting versus standing condition. During standing the ankle was in larger and more prolonged plantarflexion. This suggests that the crank-pedal angle and the position in the crank cycle where most of the effective force is applied, varies significantly from the sitting to the standing condition. Although, the knee angle during the crank cycle varies significantly from sitting to standing, the overall displacement was the same in the two conditions.
According to the literature, the standing position is likely to produce greater effective force production through peak moments involving the use of body weight as well as the pull and push of the arms through the handlebars during the downstroke and upstroke respectively. Furthermore, the cyclist tends to bring their body upward and forward over the axis of crank rotation in the standing condition thereby altering joint kinematics. The bi-articular muscles are thought to be involved in the transfer of energy from the upper limbs to the lower limbs. Additionally, the bi-articular muscles are thought to control the direction of effective force application in the standing condition.
It is likely, that the cyclist takes up the standing position to minimize the quasi-static moments which could arise if the power generating capacity of the muscles is being compromised through reduced cadence (P=F.v.). The addition of body weight and arm moments should allow the greater use of impulse (I=F.t.) and therefore momentum (M=m.v.). However, the amount of time that work (W=P.t) can be generated in the standing position is likely to be less due to greater energy expenditure. Therefore, the standing position represents a good compromise by enhancing kinematic parameters to produce greater effective pedal forces, although for a short period of time. When compared with sitting, most authors agree that the development of peak effective force and power occur later and in the region of 135-140° of the crank cycle during standing. According to the literature, this phase lag is a result of alterations in body position, as well as surface gradient incline and bicycle sideways tilt.
Clinical Case Presentations in Cyclists
- Post traumatic case presentation Jay Key - applied clinical reasoning using biomechanical principles and muscle energy techniques
- Cyclist using a fixed gear
- Pins & Needless in feet of a cyclists
Frequently Asked Questions
Pulling up?
Mr. Krause,
This is a question about working and strengthening the muscle(s) used during the recovery phase, 180 – 360 deg (approx). Last season I began slowly working on “pulling up” during the up stroke of the pedal, with the thinking that (being 56 years old) I need everything I can get to stay with the pack during a ride. At the end of the season there was tremendous improvement, and I found general use for this little bit extra during seated climbs and sprints.
Recently, I attended a 3 day bike fitting seminar, know as SICI, during which our leader who is a PhD said it wasn’t necessarily a good idea to work these muscles so much, and I could possibly cause problems if I continued. I did not argue with his position.
I’ve never had any problems with pain in any part of my leg(s) last cycling season. This winter (I’m in New England) I did leg presses at the gym and hit 500 lbs, leg extensions at 150lbs, and leg curls at 110lbs, which is not win me any prizes, but I’m not embarrassed at the end of a sprint. Also, I’m not a maniac when it comes to workouts, and I generally don’t mind some pain, but if I’m not getting paid to experience it, then I’d prefer not.
Could I please get your thoughts on this? My concern is not to cause a problem now, or later.
Sincerely,
G
Answer
Hi G,
The general wisdom is that you shouldn't pull up as you over-activate your hip flexor which can reduce blood flow into and out of the legs, alter lumbo-pelvic kinematics and create low back pain. As pedal force increases, the 'negative effective force' increases during the 'upstroke' phase (Blake et al 2012). In fact, Korff et al (2007) demonstrated reductions in gross efficiency when subjects were asked to 'pull up'. Furthermore, as power requirements increase, the time the knee spends in extension also increases (Elmer et al 2011) which would mitigate against pulling up. However, it sounds like you are using your hamstring muscles for the task. Elmer et al (2011) demonstrated increased knee flexor power as power requirements increased, effectively making the hamstrings a knee extensor.
Hence, the pulling up is fraut with danger if you have weak hamstrings or tight hip flexors (iliopsoas). Your hamies certainly don't sound weak with the leg curls and judging by your leg press you aren't lacking power there either. Hence I don't think that the loading relative to your overall strength would lead to injury, except after a period of illness or inactivity that has led to deconditioning.
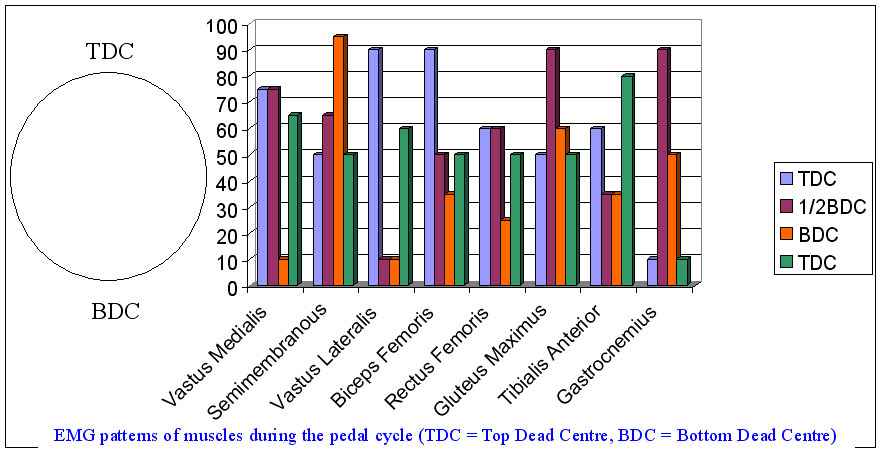
You also state you feel more power. The pulling up is important for uphill peddling and sprinting during the initial phase of getting out of the saddle. However, the hamstring is particularly more important in the downward extension phase whereby it is contracting and lengthening at the same time. Hence the elasticity and pliability of your hamies and their contractile recovery are important. Since you are going uphill I assume your cadence a little slower and hence the transition phase (lengthened muscle -> shortened) is longer. Lower cadences during higher gradients results in earlier peak vastus medialis (VM) and vastus latrealis (VL) activity earlier in the pedal cycle. Increased power output occurs for Tibialis Anterior, VM, Rectus Femoris (RF) and VL at TDC, and more soleus, semitendinosus (ST), biceps femoris (BF) and medial gastroc (GM) activity later in the downstroke (Blake & Wakeling 2012, Med Ex Sp Ex, 44, 5, 939-948). This differs to sprinting on an even gradient and hence you may need to stretch and/or massage your hamies when doing a lot of sprint training. But don't overstrech them and always stretch with a slightly bent knee (10-30 degrees) whereby you should feel the muscle pull in the middle of the hamie bulk and not at the tendons, or worse still stretching the sciatic nerve.
When you aren't powering uphill or sprinting you should focus on continuing to spin the pedals.
I hope that this helps
cheers
Martin
Uphill Training
- my personal preference for time poor cyclists is to quality hill training, whereby strength and endurance can be trained simultaneously with efficient pedalling technique
- as gradients increase to over 8% gross efficiency is lower as cadence decreases. This is compensated for by more efficient distribution of muscular force across the downward pedal cycle (Arkesteijn et al 2013, Med Sc Ex Sp, 45, 5, 920 - 926)
- slow sustained climbs involve using low gears (eg 12/13 to 53) on moderate (8%) to steep (14%) incline at a very low cadence (30rpm) for a period between 5 and 7 minutes. Total time should be in the vincinity of 21minutes (eg 3 x 7 minutes) with only a short time (4-5 minutes) for recovery.
- These slow rpms should result in some 'burning' in the leg muscles, the recognition of left - right strength deficits as well as appreciation of the upper body and core abdominal - back muscles to the overall development of synergistic strength. Hence, the cyclist should remain seated for these repeats.
- Remaining seated should mean that you are engaging the hamstring muscles to work in a synergy with the gluteal muscles and more obvious quadriceps. Importantly, if the the hamstrings are engaged correctly, there should be no sense of strain behind the knee cap (patella) or knee joint, but rather it should be felt purely within the muscle.
- Ideally, these repeates are accompanied by core stability exercises (eg 'the plank') and upper body exercises (eg japanese push-ups). Whilst doing these exercises, visualise yourself going uphill, for transference of learning to take place at a cognitive subconscious level.
- Naturally, this isn't for the faint-hearted nor should these be done in the racing season. 48-72 hours recovery between sessions is recommended. This ecovery may take the form of cycling but with low gears and preferably flat.
- The program should last 4 weeks and should be done after a 6-12 week preparatory phase of endurance/base conditioning cycling.
- After these slow sustained efforts and the basic preparatory phase, a 4 week period of pre-competition hill climbing should be undertaken 2 times per week. a rest day should preceed the first session. These efforts involve finding a hill and dividing it into halves. Where the first half is at a moderate cadence of 60-70rpm and/or 70-75% HRmax, the next half involves changing down 2 gears engaging a high cadence and high power output not exceeding 85% HRmax on 1 day per week - always stay seated if possible. 48 - 72 hours later find the same hill and divide it into thirds. First third at 75% HRmax seated, the mid third at 85% HRmax out of the saddle, and the final third 90% HRmax in week 1, 95% HRmax in week 2-3 all out effort by week 4. Generally 4- 7repeats with sessions lasting between 20 and 40minutes in total. Initially, fewer repeats to accustom oneself to the intensity and later fewer repeats due to the higher intensity i.e. weeks 2 and 3 having higher repeats. Rest day should follow the higher intensity sessions whereas the first efforts should be followed by a day of 2hours of rolling hills. This trains power.
- Depending on where you live, it would be a great idea to find a hill which lasts 45-60minutes and break it up into thirds and gradually increase the intensity each third (75% HRmax, 85% HRmax, 90% HRmax). Ideally, the incline is steady and around 8-14%. This trains strength-endurance.
- At least 1 day a week should be a long ride of 3-4hours for endurance - here some high cadence (80 - 100rpm) should probably also be employed even if it is only on the flat or downhill..
- Alternate, hill training can include changes in the variables 'incline', 'cadence', 'gear ratios', 'seated vs standing' using a fartlek methodolgy whereby recovery rate is trained through 60secs on: 60secs off for a period of 20-40minutes
- If training at altitude, there are 2 philosophies - train high : sleep low and the reverese. The concept of sleeping low is based on better hormonal recovery at night after intense training. The methodology of sleeping high and training low, is that it is easier to develop more strength during training due to less strain from lack of oxygen. Then by sleeping high, the body adapts and increases it's haemoglobin carrying capacity for oxygen, which then improves endurance. With a good endurance base, strength can be developed without risking over training and injury.
Central and Peripheral Fatigue and Central Motor Drive (CMD) as it relates to altitude training
Peripheral fatigue comprises changes in the metabolic milieu of the working muscles whereas central fatigue comprises of a failure by the central nervous system (CNS) to 'drive' the motor neurons, commonly referred to as a reduction in central motor drive (CMD). It is thought that the feedback from type III and type IV nerve receptors in muscles, about the peripheral metabolic milieu, is critical to CMD. However, in an interesting review, Markus Amann (2011, Med Sc Sp Ex, 43, 11, 2039-2045) argues that, when there is a direct threat to the CNS, as a result of adverse physiological circumstances, such as hypoglycaemia, mental stress/fatigue, or in the presence of extreme environmental influences, such as heat or severe hypoxia, these factors play a much greater influence than peripheral metabolic milieu. In the case of hypoxia, the exercising terminating threshold can be up to 30% less, when exhaustion becomes exercising limiting. Hence, when training at altitude, factors such as hypoxia and the other 'threats to the CNS' should be taken into consideration, epsecially when it comes to team training camps, where many influences out of the athletes control come into play.
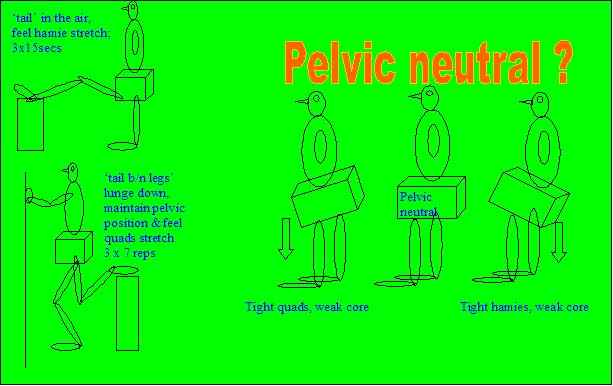
Core Stability Training using the Swiss Ball
Link to Pelvic - Neck dysfunction
Link to injuries relating to Pelvic Asymmetry
References
Blake OM, Champoux Y, Wakeling JM (2012). Muscle coordination patterns for efficient cycling. Medicine & Science in Sports & Exercise, 44, 5, 926-938
Caldwell GE, Li L, McCole SD, Hagberg JM (1998). Pedal and crank kinetics in uphill cycling. Journal of Applied Biomechanics, 14, 245 to 259.
Caldwell GE, Hagberg JM, McCole SD, Li L (1999). Lower extremity joint moments during uphill cycling. Journal of Applied Biomechanics, 15, 166 to 181.
Cavanagh PR, Sanderson DJ (1986). The biomechanics of cycling: Studies of the pedaling mechanics of elite pursuit riders. In : Science of Cycling, Human Kinetics Books, Champaign, ch5.
Davison RCR, Swan D, Coleman D, Bird S (2000). Correlates of simulated hill climb cycling performance. Journal of Sports Sciences, 18, 105 to 110
Fregly BJ, Zajac FE (1996) A state-space analysis of mechanical energy generation, absorption, and transfer during pedaling. Journal of Biomechanics, 29, 1, 81 to 90
Gregor RJ Fowler E (1996). Biomechanics of Cycling. In : Zachazewski JE, Magee DJ, Quillen WS. Athletic Injuries and Rehabilitation, WB Saunders Co, Philadelphia, Ch19
Hodson-Tole EF, Blake OM, Wakeling JM (2020) During cycling what limits maximum mechanical power output at cadences above 120rpm. Med Sc Sp Ex, 52, 1, 214-224
Jones DA, Round JM (1990). Skeletal muscle in health and disease. Manchester, Manchester University Press pp24 to 25.
Li l, Caldwell GE (1998) Muscle co-ordination in cycling: effect of surface incline and posture. Journal Applied Physiology, 85, 3, 927 to 934.
Lieber RL (1992). Skeletal muscle structure and function. Williams & Wilkins, Baltimore, pp41 to 42
Price D, Donne B (1997) Effect of variation in seat tube angle at different seat heights on submaximal cycling performance in man. Journal of Sports Science, 15, 395 to 402.
Rugg SG, Gregor RJ (1987) The effect of seat height on muscle lengths, velocities and moment arm lengths during cycling. Journal of Biomechanics, 20, 899
Ryschon TW, Stray-Gundersen, J (1991). The effect of body position on the energy cost of cycling. Medicine and Science in Sports and Exercise, 23, 949 to 953.
Shemmell JB, Neal RJ (1998) The kinematics of uphill, out of the saddle cycling. The North American Congress on Biomechanics, August 14-18, University of Waterloo, Ontario, Canada
Soderberg GL (1992). Skeletal muscle function. In : Currier DP, Nelson RM (1992) Dynamics of Human Biological Tissue, FA Davis Company, Philadelphia, Ch 3.
Stone C, Hull ML (1993). Rider/bicycle interaction loads during standing treadmill cycling. Journal of Applied Biomechanics, 9, 202 to 218.
Too D (1996) The kinematics of uphill cycling. Proceedings 9th CSB biennial conference, 184 to 185.
Too D, Lndwer GE (2000) The effect of pedal crank arm length on joint angle and power production in upright cycle ergometry. Journal of Sports Sciences, 18, 153 to 161
Van Ingen Schenau GJ (1989). From rotation to translation: constraints on multi-joint movements and the unique action of biarticular muscles. Human movement sciences, 8, 301 to 337.
Van Ingen Schenau GJ, Boots PJM, de Groot G, Snackers RJ, van Woensel WWLM (1992). The constrained control of force and position in multi-joint movements. Neuroscience, 46, 197 to 207.
Winter EM, Brown D, Roberts NKA, Brookes FBC, Swaine IL (1996). Optimized and corrected peak power output during friction-braked cycle ergometry. Journal of Sports Sciences, 14, 513 to 521.
First Upload : 2004
Last update : 25 February 2020